Antenna Arrays

A weighting method is a means of selecting the weights that multiply the signals from the antennas in an antenna array: The weights used in the antenna array are fundamental in controlling the behavior of the array. Some methods are now presented, which also serve to explain the versatility of antenna arrays. Phased Arrays Schelkunoff Polynomial Method (Null Placement) Dolph-Tschebysheff Weights MMSE Weights Adaptive Antenna Arrays: The LMS Algorithm Antenna Arrays Home Antenna Home Phased Array Weighting in Antenna Arrays If a plane wave is incident upon an antenna array (Figure 1), the phase of the signal at the antennas will be a function of the angle of arrival of the plane wave. If the signals are then added together, they may add constructively or destructively, depending on the phases. 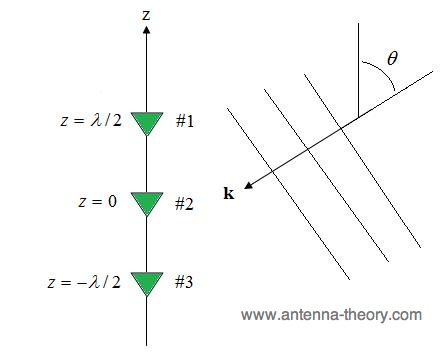Figure 1. The phase of the signal at each element depends on the angle of arrival of the plane wave. Let's say that we wanted to receive signals from an angle of 45 degrees, as in Figure 1. In that case, we would have a good idea of what the phases will be across the antenna array. Suppose that we multiplied the signal from each antenna by a complex phase () that cancelled out the phase change due to the propagation of the wave. Then when the signals from each antenna are added together to form the output of the array, they would combine coherently. This is the fundamental principle used in phased arrays - also known as beam steering. Let's take an example. Suppose that we had a N=5 element linear array with one-half wavelength spacing between the antennas (assumed to be isotropic for simplicity). The positions are each given by: for n = 0,1,2,3,4. If we want the array to be steered towards 45 degrees (), then weights can be applied that are given by: To be absolutely explicit about it, the weight vector can also be written as: The resulting Array Factor for this array becomes: In the above, k is the wave vector, v(k) is the steering vector, and N=5. The array response is the magnitude of the output, as a function of the incident angle of the plane wave. The array response is identical in form to the radiation pattern of this array (when the same weights are used). This response is shown in Figure 2. Figure 2. Response of array versus angle of incidence of a plane wave. Figure 2 captures the fundamental properties of the designed array. First, the array has maximum radiation (or reception) in the direction of (img src="thetad.jpg">), as designed. Second, the array has nulls at roughly 72, 94, 120, and 153 degrees. The null-directions are those in which the array completely blocks signals. This array has an associated HPBW of roughly 30 degrees. Finally, note that the sidelobes are 12 dB below the peak of the main beam. Using a phased-weighting scheme is the simplest of all weighting methods. By employing this method, the array can be steered such that the direction of maximum reception is in a desired direction. The ease of implementation is responsible for its widespread use; however, as we'll see, there are better methods of steering (although the complexity increases). Next: Grating Lobes in Phased Arrays Up: Antenna Arrays (Home) Antennas (Home) This page on the phased array weighting method is copyrighted. No portion can be reproduced or copied except by permission of the author. Copyright antenna-theory.com, 2009, 2010, 2011. Phased array weighting weights.